

The nth term of an arithmetic sequence with an initial term A sub one and a common difference D, is A sub N equals A sub one plus N minus one times D. We symbolize the common difference with the letter D. Because if we subtract any two adjacent terms, we get this same number. The fixed amount we add each time to get each term is called the common difference. We're familiar with that from the last video. First of all, the starting term in general's a sub 1. So that means that the nth term of this sequence would be 5 plus 7 times n minus 1. Of course, a compact way to add n minus 1 individual 7's would simply be to multiply 7 times n minus 1. Thus, the nth term, a sub n, would be the starting term plus n minus one individual sevens. In each term the number of seven's added as one less than the index number of the term. It's one less than the number on the list. Notice that the number of sevens, in each row, the number of sevens that we're adding is one less than the index number. And so what we have here, this stream of sevens. Then the fourth term, we add seven again, then add seven again. So I'm gonna write that as five plus seven plus seven. To get the third term, we add seven again.
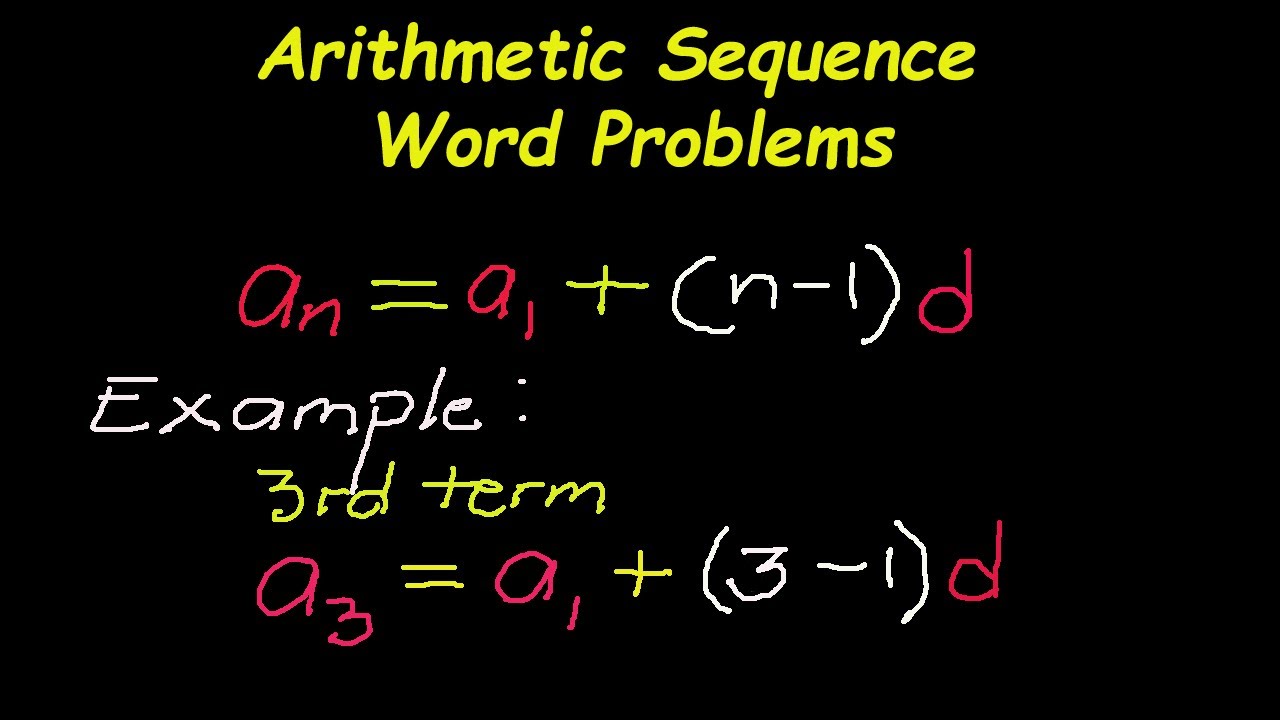

The sequence that starts with five, and then we add seven each time. So I'll focus on this particular sequence. I'm gonna analyze the individual terms in the following way to look for a pattern. But we'd like a formula that would allow us to do that for arithmetic sequences. If the test said find the 80th term in this sequence, all we'd have to do is plug in. Remember for the algebraically defined sequences that we saw in the previous video, it's very easy. It would be helpful to find a formula for the nth term, so that, for example, we could find the value of a much higher term in the sequence. Consecutive odd numbers, consecutive even numbers, or consecutive integers are also special cases of arithmetic sequences. We would add p to get from each term to the next. One special case is consecutive multiples of an integer p. Any evenly spaced list is an arithmetic sequence. So we could continue this pattern forever, just continually adding seven to get the next number. That's how we go from one term to the next. So if you look at this sequence, you'll notice that to get from each term to the next, we're simply adding seven. An arithmetic sequence is one in which we add the same constant to get from each term to the next. One special kind of sequence is called an arithmetic sequence.


 0 kommentar(er)
0 kommentar(er)
